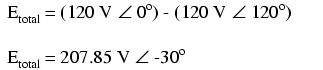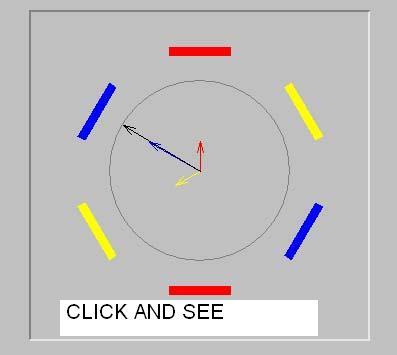
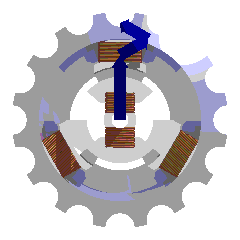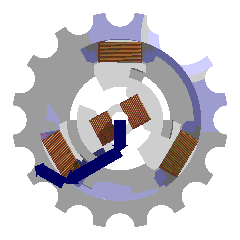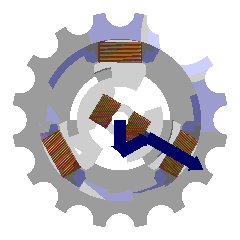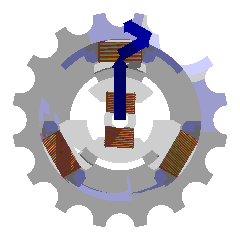
Mode triphasé On considère trois paires de bobines plates identiques équidistantes de l'axe du système et dont les axes font entre eux des angles de 120°. Elles sont parcourues par les courants : I1 = Io.cos(wt) I2 = Io.cos(wt + 2p/3) I3 = Io.cos(wt + 4p/3) (Courant triphasé) Dans la simulation, on trace l'évolution au cours du temps de l'induction magnétique due à chaque bobine (trait de la couleur des bobines correspondantes) ainsi que l'induction résultante (trait noir). Le module du vecteur B reste constant mais ce vecteur tourne autour de O à la vitesse constante wt. C'est sur ce principe que sont basés les moteurs triphasés. Dans chaque cas, vous pouvez modifier la fréquence de rotation,  geler l'animation et modifier le sens de la rotation du champ résultant.  Pour ce faire, on permute les courants dans les bobines 1 et 2. 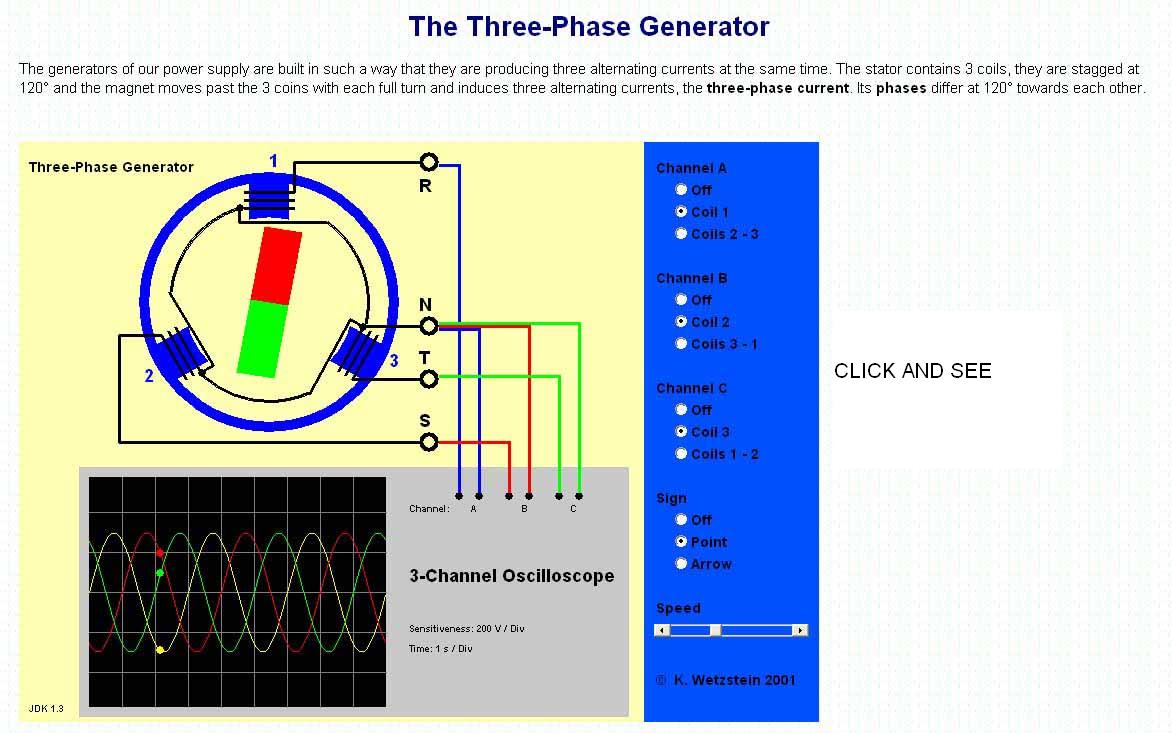http://www.univ-lemans.fr/enseignements/physique/02/electri/triphase.html http://www.k-wz.de/physik/threephasegenerator.html The Three-Phase Generator The generators of our power supply are built in such a way that they are producing three alternating currents at the same time. The stator contains 3 coils, they are stagged at 120° and the magnet moves past the 3 coins with each full turn and induces three alternating currents, the three-phase current. Its phases differ at 120° towards each other.   From Wikipedia, the free encyclopedia   Jump to: navigation, search Three-phase power transformer which is the sole transfer point for electricity to a suburban shopping mall in Canada. Note the four wires used for the 208 V/120Y service: one is for the neutral, and the other three are for the X, Y, and Z phases. Three Phase Electric Power Transmission Three-phase is a common method of electric power transmission. It is a type of polyphase system used to power motors and many other devices. This article deals with where, how and why "three phase" is used. For information on the basic mathematics and principles of three phase see three-phase. For information on testing three phase equipment (kit) please see three-phase testing. Three phase systems may or may not have a neutral wire. A neutral wire allows the three phase system to use a higher voltage while still supporting lower voltage single phase appliances. In high voltage distribution situations it is common not to have a neutral wire as the loads can simply be connected between phases (phase-phase connection). Three phase has properties that make it very desirable in electric power systems. Firstly the phase currents tend to cancel one another (summing to zero in the case of a linear balanced load). This makes it possible to eliminate the neutral conductor on some lines. Secondly power transfer into a linear balanced load is constant, which helps to reduce generator and motor vibrations. Finally, three-phase systems can produce a magnetic field that rotates in a specified direction, which simplifies the design of electric motors. Three is the lowest phase order to exhibit all of these properties. Most domestic loads are single phase. Generally three phase power either does not enter domestic houses at all, or where it does, it is split out at the main distribution board. The three phases are typically indicated by colors which vary by country. See the table for more information. Contents [hide] 1 Generation and distribution 2 Single-phase loads 3 Connecting phase-to-phase 4 Three-phase loads 5 Phase converters 6 Small scale applications 7 Alternatives to three-phase 8 Color codes 9 See also 10 External links   [edit]   Generation and distribution Condiitions for connections of two generators same rotating phases same frequence same voltage phases inside of a defined window at closing Animation of three-phase power flow At the power station, an electrical generator converts mechanical power into a set of alternating electric currents, one from each electromagnetic coil or winding of the generator. The currents are sinusoidal functions of time, all at the same frequency but with different phases. In a three-phase system the phases are spaced equally, giving a phase separation of 120°. The frequency is typically 50 Hz in Europe and 60 Hz in the US and Canada (see List of countries with mains power plugs, voltages and frequencies). Generators output at a voltage that ranges from hundreds of volts to 30,000 volts. At the power station, transformers "step-up" this voltage to one more suitable for transmission. After numerous further conversions in the transmission and distribution network the power is finally transformed to the standard mains voltage (i.e. the "household" voltage). The power may already have been split into single phase at this point or it may still be three phase. Where the stepdown is 3 phase, the output of this transformer is usually star connected with the standard mains voltage (120 V in North America and 230 V in Europe) being the phase-neutral voltage. Another system commonly seen in North America is to have a delta connected secondary with a centre tap on one of the windings supplying the ground and neutral. This allows for 240 V three phase as well as three different single phase voltages (120 V between two of the phases and the neutral, 208 V between the third phase (known as a wild leg) and neutral and 240 V between any two phases) to be made available from the same supply. [edit]   Single-phase loads Single-phase loads may be connected to a three-phase system, either by connecting across two live conductors (a phase-to-phase connection), or by connecting between a phase conductor and the system neutral, which is either connected to the center of the Y (star) secondary winding of the supply transformer, or is connected to the center of one winding of a delta transformer (Highleg Delta system). (see Transformer#Polyphase transformers and Split phase ) Single-phase loads should be distributed evenly between the phases of the three-phase system for efficient use of the supply transformer and supply conductors. The line-to-line voltage of a three-phase system is √3 times the line to neutral voltage. Where the line-to-neutral voltage is a standard utilization voltage, (for example in a 240 V/415 V system) individual single-phase utility customers or loads may each be connected to a different phase of the supply. Where the line-to-neutral voltage is not a common utilization voltage, for example in a 347/600 V system, single-phase loads must be supplied by individual step-down transformers. In multiple-unit residential buildings in North America, lighting and convenience outlets can be connected line-to-neutral to give the 120 V utilization voltage, and high-power loads such as cooking equipment, space heating, water heaters, or air conditioning can be connected across two phases to give 208 V. This practice is common enough that 208 V single-phase equipment is readily available in North America. Attempts to use the more common 120/240 V equipment intended for three-wire single-phase distribution may result in poor performance since 240 V heating equipment will only produce 75% of its rating when operated at 208 V. Where three phase at low voltage is otherwise in use, it may still be split out into single phase service cables through joints in the supply network or it may be delivered to a master distribution board (breaker panel) at the customer's premises. Connecting an electrical circuit from one phase to the neutral generally supplies the country's standard single phase voltage (120 VAC or 230 VAC) to the circuit. The power transmission grid is organized so that each phase carries the same magnitude of current out of the major parts of the transmission system. The currents returning from the customers' premises to the last supply transformer all share the neutral wire, but the three-phase system ensures that the sum of the returning currents is approximately zero. The delta wiring of the primary side of that supply transformer means that no neutral is needed in the high voltage side of the network. [edit]   Connecting phase-to-phase Connecting between two phases provides √3 or 173% of the single-phase voltage (208 VAC in US; 400 VAC in Europe) because the out-of-phase waveforms add to provide a higher peak voltage in the resulting waveform. Such connection is referred to as a line to line connection and is usually done with a two-pole circuit breaker. This kind of connection is typically used for high-power appliances, because it can provide nearly twice as much power for the same current. This allows more power to be supplied for a given wire size. This may also allow loads to be served that would otherwise be so large as to exceed the capability of the building's wiring. Existing wiring can be reconnected to provide the higher voltage to the load. In USA, for example, where the single-phase voltage is 120 V, a 2 kW 208 volt electric baseboard heater could require use of a phase-phase connection where only standard wiring exists. (Note that electrical codes typically would require wire color coding to be readjusted in this case.) [edit]   Three-phase loads The rotating magnetic field of a three-phase motor. The most important class of three-phase load is the electric motor. A three phase induction motor has a simple design, inherently high starting torque, and high efficiency. Such motors are applied in industry for pumps, fans, blowers, compressors, conveyor drives, and many other kinds of motor-driven equipment. A three-phase motor will be more compact and less costly than a single-phase motor of the same voltage class and rating; and single-phase AC motors above 10 HP (7.5 kW) are uncommon. Three phase motors will also vibrate less and hence last longer than single phase motor of the same power used under the same conditions. Large air conditioning equipment (for example, most York units above 2.5 tons (8.8 kW) cooling capacity) use three-phase motors for reasons of efficiency , economy and longevity. Resistance heating loads such as electric boilers or space heating may be connected to three-phase systems. Electric lighting may also be similarly connected. These types of loads do not require the revolving magnetic field characteristic of three-phase motors but take advantage of the higher voltage and power level usually associated with three-phase distribution. Large rectifier systems may have three-phase inputs; the resulting DC current is easier to filter (smooth) than the output of a single-phase rectifier. Such rectifiers may be used for battery charging, electrolysis processes such as aluminum production, or for operation of DC motors. An interesting example of a three-phase load is the electric arc furnace used in steelmaking and in refining of ores. In much of Europe stoves are designed to allow for a three phase feed. Usually the individual heating units are connected between phase and neutral to allow for connection to a single phase supply where this is all that is available. [edit]   Phase converters Occasionally the advantages of three-phase motors make it worthwhile to convert single-phase power to three phase. Small customers, such as residential or farm properties may not have access to a three-phase supply, or may not want to pay for the extra cost of a three-phase service, but may still wish to use three-phase equipment. Such converters may also allow the frequency to be varied allowing speed control. Some locomotives are moving to multi-phase motors driven by such systems even though the incoming supply to a locomotive is nearly always either DC or single phase AC. Because single-phase power is interrupted at each moment that the voltage crosses zero but three-phase delivers power continuously, any such converter must have a way to store energy for the necessary fraction of a second. One method for using three-phase equipment on a single-phase supply is with a rotary phase converter, essentially a three-phase motor with special starting arrangements and power factor correction that produces balanced three-phase power. When properly designed these rotary converters can allow satisfactory operation of three-phase equipment such as machine tools on a single phase supply. In such a device, the energy storage is performed by the mechanical inertia (flywheel effect) of the rotating components. Another method often attempted is with a device referred to as a static phase converter. This method of running three phase equipment is commonly attempted with motor loads though it only supplys 2/3 power and can cause the motor loads to run hot and in some cases overheat. This method will not work when any circuitry is involved such as cnc devices, or in induction and rectifier type loads. Some devices are made which create an imitation three-phase from three-wire single phase supplies. This is done by creating a third "subphase" between the two live conductors, resulting in a phase separation of 180° − 90° = 90°. Many three-phase devices will run on this configuration, but at lower efficiency. It can be valuable to look up the various ratings of 3 phase converter technology with the US Phase Converter Standards Organization. They regulate the standards of phase converters manufactured in the US and provide ratings on various technologies used to convert single phase power to three phase power. Variable frequency drives (also known as solid-state inverters) are used to provide precise speed and torque control of three phase motors. Some models can be powered by a single phase supply. VFDs work by converting the supply voltage to DC and converting the DC to a suitable three phase source for the motor. The drives usually include large capacitors to smooth out supply variations and zero crossing states. [edit]   Small scale applications While most three-phase motors are very big (>750w), there are small (<50w) three-phase motors. The most common example is a computer fan. An inverter circuit inside the fan converts DC to three-phase AC. This is done to decrease noise (as the torque from a three-phase motor is very smooth compared to that from a single phase motor or a brushed DC motor) and increase reliability (as there are no brushes to wear out, unlike a brushed DC motor). [edit]   Alternatives to three-phase Three-wire single-phase distribution is useful when high voltage three phase is not available, and allows double the normal utilization voltage to be supplied for high-power loads. Two phase power, like three phase, gives constant power transfer to a linear load. For loads which connect each phase to neutral, assuming the load is the same power draw , the two wire system has a neutral current which is greater than neutral current in a three phase system. Also motors aren't entirely linear and this means that despite the theory motors running on three phase tend to run smoother than those on two phase. The generators at Niagara Falls installed in 1895 were the largest generators in the world at the time and were two-phase machines. True two-phase power distribution is essentially obsolete. Special purpose systems may use a two-phase system for control. Two-phase power may be obtained from a three-phase system using an arrangement of transformers called a Scott T. Monocyclic power was a name for an asymmetrical modified two-phase power system used by General Electric around 1897 (championed by Charles Proteus Steinmetz and Elihu Thomson; this usage was reportedly undertaken to avoid patent legalities). In this system, a generator was wound with a full-voltage single phase winding intended for lighting loads, and with a small (usually 1/4 of the line voltage) winding which produced a voltage in quadrature with the main windings. The intention was to use this "power wire" additional winding to provide starting torque for induction motors, with the main winding providing power for lighting loads. After the expiration of the Westinghouse patents on symmetrical two-phase and three-phase power distribution systems, the monocyclic system fell out of use. High phase order systems for power transmission have been built and tested. Such transmission lines use 6 or 12 phases and design practices characteristic of extra-high voltage transmission lines. High-phase order transmission lines may allow transfer of more power through a given transmission line right-of-way without the expense of a HVDC converter at each end of the line. [edit]   Color codes Conductors of a three phase system are usually identified by a color code, to allow for balanced loading and to assure the correct phase rotation for induction motors. Colors used may adhere to old standards or to no standard at all, and may vary even within a single installation. However, the current National Electrical Code (2005) does not require any color identification of conductors other than that of the neutral (white or white with a color stripe), the ground (green or green with a yellow stripe), and, in the case of a High Leg Delta system, the High Leg ("shall be durably and permanently marked by an outer finish that is orange in color or by other effective means"). (NEC 110.15).   L1 L2 L3 Neutral Ground North America Black Red Blue White Green North America (newer 277/480 installations) Brown Orange Yellow White Green UK until April 2006 (colours in brackets are Harmonised colours) Red (Brown) Yellow (prev. white) (Black) Blue (Grey) Black (Blue) Green/yellow striped (green on very old installations, approx. before 1970) Europe (including UK) from April 2004 Brown Black Grey Blue Green/yellow striped Previous European (varies by country) Brown or black Black or brown Black or brown Blue Green/yellow striped Europe, for busbars Yellow Green Purple – – Australia Red White (prev. yellow) Blue Black Green/yellow striped (green on very old installations) Note that in the U.S. a green/yellow striped wire typically indicates an Isolated ground.

	THE COLOURS DO NOT CORRESPOND LEFT AND RIGHT BLEUE IS OK RED LEFT IS BLACK RIGHT YELLOW LEFT IS RED RIGHT
DIRECT PHASES ROTATION

INVERSE PHASES ROTATION	PARALLELE OPERATION FORBIDDEN	SYNCHRONISING AND CONNECTION GIVE A SHORT CIRCUIT

Variable setup and basic definitions

One voltage cycle of a three-phase system, labelled 0 to 360° ( 2 π radians) along the time axis. The plotted line represents the variation of instantaneous voltage (or current) with respect to time. This cycle will repeat 50 or 60 times per second, depending on the power system frequency. The colours of the lines represent the American color code for three-phase. That is black=V_L1 red=V_L2 blue=V_L3

Let

where t is time and f is frequency.

Using x = ft the waveforms for the three phases are

where A is the peak voltage and the voltages on L1, L2 and L3 are measured relative to the neutral.

Balanced loads

Generally, in electric power systems the loads are distributed as evenly as practical between the phases. It is usual practice to discuss a balanced system first and then describe the effects of unbalanced systems as deviations from the elementary case.

To keep the calculations simple we shall normalise A and R to 1 for the remainder of these calculations

Star connected systems with neutral

Constant power transfer

An important property of three-phase power is that the power available to a resistive load, , is constant at all times.

Using : R = 1 and A = 1

Using angle subtraction formulae

Using the Pythagorean trigonometric identity

since we have eliminated x we can see that the total power does not vary with time. This is essential for keeping large generators and motors running smoothly.

No neutral current

The neutral current is the sum of the phase currents.

Using : R = 1 and A = 1

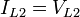
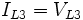
Using angle subtraction formulae

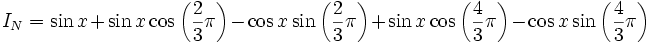
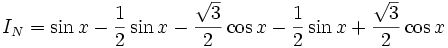
Star connected systems without neutral

Since we have shown that the neutral current is zero we can see that removing the neutral core will have no effect on the circuit, provided the system is balanced. In reality such connections are generally used only when the load on the three phases is part of the same piece of equipment (for example a three-phase motor), as otherwise switching loads and slight imbalances would cause large voltage fluctuations.

Three-Phase

Three-phase AC is not hard to understand if you use a phasor diagram

Most alternating-current (AC) generation and transmission, and a good part of use, take place through three-phase circuits. If you want to understand electric power, you must know something about three-phase. It is rather simple if you go at it the right way, though it has a reputation for difficulty.

Phase is a frequently-used term around AC. The word comes from Greek fasis, "appearance," from fanein, "to appear." It originally referred to the eternally regular changing appearance of the moon through each month, and then was applied to the periodic changes of some quantity, such as the voltage in an AC circuit. Electrical phase is measured in degrees, with 360° corresponding to a complete cycle. A sinusoidal voltage is proportional to the cosine or sine of the phase.

Three-phase, abbreviated 3φ, refers to three voltages or currents that that differ by a third of a cycle, or 120 electrical degrees, from each other. They go through their maxima in a regular order, called the phase sequence. The three phases could be supplied over six wires, with two wires reserved for the exclusive use of each phase. However, they are generally supplied over only three wires, and the phase or line voltages are the voltages between the three possible pairs of wires. The phase or line currents are the currents in each wire. Voltages and currents are usually expressed as rms or effective values, as in single-phase analysis.

When you connect a load to the three wires, it should be done in such a way that it does not destroy the symmetry. This means that you need three equal loads connected across the three pairs of wires. This looks like an equilateral triangle, or delta, and is called a delta load. Another symmetrical connection would result if you connected one side of each load together, and then the three other ends to the three wires. This looks like a Y, and is called a wye load. These are the only possibilities for a symmetrical load. The center of the Y connection is, in a way, equidistant from each of the three line voltages, and will remain at a constant potential. It is called the neutral, and may be furnished along with the three phase voltages. The benefits of three-phase are realized best for such a symmetrical connection, which is called balanced. If the load is not balanced, the problem is a complicated one one whose solution gives little insight, just numbers. Such problems are best left to computer circuit analysis. Three-phase systems that are roughly balanced (the practical case) can be analyzed profitably by a method called symmetrical components. Here, let us consider only balanced three-phase circuits, which are the most important anyway.

The key to understanding three-phase is to understand the phasor diagram for the voltages or currents. In the diagram at the right, a, b and c represent the three lines, and o represents the neutral. The red phasors are the line or delta voltages, the voltages between the wires. The blue phasors are the wye voltages, the voltages to neutral. They correspond to the two different ways a symmetrical load can be connected. The vectors can be imagined rotating anticlockwise with time with angular velocity ω = 2πf, their projections on the horizontal axis representing the voltages as functions of time. Note how the subscripts on the V's give the points between which the voltage is measured, and the sign of the voltage. V_ab is the voltage at point a relative to point b, for example. The same phasor diagram holds for the currents. In this case, the line currents are the blue vectors, and the red vectors are the currents through a delta load. The blue and red vectors differ in phase by 30°, and in magnitude by a factor of √3, as is marked in the diagram.

Suppose we want to take two phase wires and neutral to make a three-wire household service supplying 120 V between each hot wire and ground. The neutral will become the grounded conductor, the two phases the hot conductors. Then, the wye voltage is 120, so the delta voltage will be √3 x 120 = 208 V. This is the three-phase line voltage necessary in this case. Note that the two 120 V sources are not opposite in phase, and will not give 240 V between them. On the other hand, suppose we do want a 240 V service. Then this must be the line voltage, and the voltages to neutral will be 139 V, not 120 V. A 120 V three-phase service will give only 69 V from line to neutral. Note that √3 appears everywhere, and that the differences in phase explain the unexpected results.

If the load consists of general impedances Z, the situation is described by current and voltage phasors connected by V = IZ, both in magnitude and phase. The diagrams are similar in shape, and rotated by the phase angle between voltage and current in each impedance. Remember that the line voltages are the red vectors, while the line currents are the blue vectors. Z relates either the line voltages and delta currents, or the wye voltages and the line currents, depending on the connection. Z does not relate the line current and line voltage, which are different in phase by 30° even for unity power factor (pure resistance load).

This comes out more clearly when we consider the power P delivered to the load. For a resistive delta load, P = 3 V_lineI_delta = √3 V_lineI_line, since I_delta = √3 I_line. For a wye load, P = 3 V_wyeI_line = √3 V_lineI_line. This is, of course, the same expression. For other than unity power factor, this must be multiplied by cos θ, which is the angle of Z, not the phase difference between the line voltage and line current. This means, most emphatically, that our usual rule for finding the power from phasors does not apply to three-phase!

If you write out the three phase currents as explicit functions of time, I_maxcos ωt, I_maxcos (ωt - 120°) and I_maxcos (ωt + 120°), square them, multiply by the resistance R, and add, the result is the constant (3/2)I_max²R = 3 I²R. The power is applied steadily as in DC circuits, not in pulses as in single-phase AC circuits. This is a great advantage, giving three-phase machines 48% greater capacity than identical single-phase machines.

In Germany and Switzerland, where three-phase power was originated and developed, it is known as Drehstrom, "rotating current" for this property of constant power. Ordinary AC is called Wechselstrom, or "change current." Nikola Tesla, the discoverer of polyphase currents and inventor of the induction motor, employed two-phase current, where the phase difference is 90°. This also can be used to create a rotating magnetic field, and is more efficient than single-phase, but is not quite as advantageous as three-phase. Two-phase power was once rather common in the United States, where Tesla was important in the introduction of AC, but has now gone completely out of use.

Two-phase can be supplied over three wires, but there is no true neutral, since the phases are not symmetrical. However, it is always easy to double the number of phases in a transformer secondary by making two secondary windings and connecting them in opposing phases. Four-phase does have a neutral, like three-phase, but requires four wires. In fact, three-phase is more economical than any other number of phases. For applications like rectifiers and synchronous converters where DC is produced, it is most efficient to use six-phase AC input, which is easily produced from three-phase in a transformer.

If you are transmitting a certain amount of power single-phase, adding one more conductor operated at the same line voltage and current and using three-phase will increase the power transmitted by 72% with only a 50% increase in the amount of copper and losses. The advantage is obvious. Under certain conditions, transmitting a certain amount of power by three-phase only requires 75% of the copper of single-phase transmission. This is not the major advantage of three-phase, but it does play a factor.

Three wires are usually seen in high-voltage transmission lines, whether on towers or poles, with pin or suspension insulators. Some high-voltage lines are now DC, since solid state devices make it easier to convert to and from AC. The DC lines are free of the problems created by phase, as well as eliminating the skin effect that reduces the effective area of the conductors. It is not nearly as easy to manage long-distance electrical transmission as might be thought.

Unbalanced systems

Practical systems rarely have perfectly balanced loads, currents, voltages or impedances in all three phases. The analysis of unbalanced cases is greatly simplified by the use of the techniques of symmetrical components. An unbalanced system is analyzed as the superposition of three balanced systems, each with the positive, negative or zero sequence of balanced voltages.

Revolving magnetic field

Any polyphase system, by virtue of the time displacement of the currents in the phases, makes it possible to easily generate a magnetic field that revolves at the line frequency. Such a revolving magnetic field makes polyphase induction motors possible. Indeed, where induction motors must run on single-phase power (such as is usually distributed in homes), the motor must contain some measure to produce a revolving field, otherwise the motor cannot generate any stand-still torque and will not start. The field produced by a single-phase winding can provide energy to a motor already rotating, but without auxiliary functions the motor will not accelerate from a stop when energized.

Conversion to other phase systems

Provided two voltage waveforms have at least some relative displacement on the time axis, other than a multiple of a half-cycle, any other polyphase set of voltages can be obtained by an array of passive transformers. Such arrays will evenly balance the polyphase load between the phases of the source system. For example, balanced two-phase power can be obtained from a three-phase network by using two specially constructed transformers, with taps at 50% and 86.6% of the primary voltage. This Scott T connection produces a true two-phase system with 90° time difference between the phases. Another example is the generation of higher-phase-order systems for large rectifier systems, to produce a smoother DC output and to reduce the harmonic currents in the supply.

When three-phase is needed but only single-phase is readily available from the utility company a phase converter can be used to generate three-phase power from the single phase supply. The US Phase Converter Standards Organization conducts independent three phase tests on the various phase converter technologies and publishes the results.

If the frequency (HZ) of the three-phase power supplied does not match the frequency needed to run the machines or equipment a Frequency converter can be used

Home Guided tour   Wind     Turbine siting     Energy output     How does it work?     Generators     Turbine design     Manufacturing     R & D     Electrical grid     Environment     Economics     History of wind energy     Wind energy manual         Index         Wind energy concepts         Energy and power         Proof of Betz' law         Wind turbine acoustics         Electricity         3 phased electricity         3 phased connection         Electromagnetism 1         Electromagnetism 2         Induction 1         Induction 2         Environment and fuels         Bibliography         Glossary		3 Phase Alternating Current The power of alternating current (AC) fluctuates. For domestic use for e.g. light bulbs this is not a major problem, since the wire in the light bulb will stay warm for the brief interval while the power drops. Neon lights (and your computer screen) will blink, in fact, but faster than the human eye is able to perceive. For the operation of motors etc. it is useful, however, to have a current with constant power. Voltage Variation for Three Phase Alternating Current It is indeed possible to obtain constant power from an AC system by having three separate power lines with alternating current which run in parallel, and where the current phase is shifted one third of the cycle, i.e. the red curve above is running one third of a cycle behind the blue curve, and the yellow curve is running two thirds of a cycle behind the blue curve. As we learned on the previous page, a full cycle lasts 20 milliseconds (ms) in a 50 Hz grid. Each of the three phases then lag behind the previous one by 20/3 = 6 2/3 ms. Wherever you look along the horizontal axis in the graph above, you will find that the sum of the three voltages is always zero, and that the difference in voltage between any two phases fluctuates as an alternating current. On the next page you will see how we connect a generator to a three phase grid.
		© Copyright 1997-2003 Danish Wind Industry Association Updated 12 May 2003 http://www.windpower.org/en/stat/unitsac3.htm

Three-phase Y and Δ configurations

Three-phase Y and Δ configurations

Initially we explored the idea of three-phase power systems by connecting three voltage sources together in what is commonly known as the "Y" (or "star") configuration. This configuration of voltage sources is characterized by a common connection point joining one side of each source:

If we draw a circuit showing each voltage source to be a coil of wire (alternator or transformer winding) and do some slight rearranging, the "Y" configuration becomes more obvious:

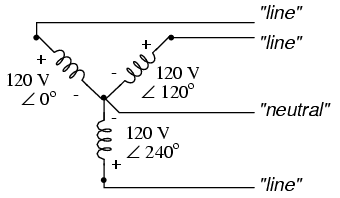
The three conductors leading away from the voltage sources (windings) toward a load are typically called lines, while the windings themselves are typically called phases. In a Y-connected system, there may or may not be a neutral wire attached at the junction point in the middle, although it certainly helps alleviate potential problems should one element of a three-phase load fail open, as discussed earlier:

When we measure voltage and current in three-phase systems, we need to be specific as to where we're measuring. Line voltage refers to the amount of voltage measured between any two line conductors in a balanced three-phase system. With the above circuit, the line voltage is roughly 208 volts. Phase voltage refers to the voltage measured across any one component (source winding or load impedance) in a balanced three-phase source or load. For the circuit shown above, the phase voltage is 120 volts. The terms line current and phase current follow the same logic: the former referring to current through any one line conductor, and the latter to current through any one component.

Y-connected sources and loads always have line voltages greater than phase voltages, and line currents equal to phase currents. If the Y-connected source or load is balanced, the line voltage will be equal to the phase voltage times the square root of 3:

However, the "Y" configuration is not the only valid one for connecting three-phase voltage source or load elements together. Another configuration is known as the "Delta," for its geometric resemblance to the Greek letter of the same name (Δ). Take close notice of the polarity for each winding in the drawing below:

At first glance it seems as though three voltage sources like this would create a short-circuit, electrons flowing around the triangle with nothing but the internal impedance of the windings to hold them back. Due to the phase angles of these three voltage sources, however, this is not the case.

One quick check of this is to use Kirchhoff's Voltage Law to see if the three voltages around the loop add up to zero. If they do, then there will be no voltage available to push current around and around that loop, and consequently there will be no circulating current. Starting with the top winding and progressing counter-clockwise, our KVL expression looks something like this:

Indeed, if we add these three vector quantities together, they do add up to zero. Another way to verify the fact that these three voltage sources can be connected together in a loop without resulting in circulating currents is to open up the loop at one junction point and calculate voltage across the break:

Starting with the right winding (120 V ∠ 120^o) and progressing counter-clockwise, our KVL equation looks like this:

Sure enough, there will be zero voltage across the break, telling us that no current will circulate within the triangular loop of windings when that connection is made complete.

Having established that a Δ-connected three-phase voltage source will not burn itself to a crisp due to circulating currents, we turn to its practical use as a source of power in three-phase circuits. Because each pair of line conductors is connected directly across a single winding in a Δ circuit, the line voltage will be equal to the phase voltage. Conversely, because each line conductor attaches at a node between two windings, the line current will be the vector sum of the two joining phase currents. Not surprisingly, the resulting equations for a Δ configuration are as follows:

Let's see how this works in an example circuit:

With each load resistance receiving 120 volts from its respective phase winding at the source, the current in each phase of this circuit will be 83.33 amps:

So, the each line current in this three-phase power system is equal to 144.34 amps, substantially more than the line currents in the Y-connected system we looked at earlier. One might wonder if we've lost all the advantages of three-phase power here, given the fact that we have such greater conductor currents, necessitating thicker, more costly wire. The answer is no. Although this circuit would require three number 1 gage copper conductors (at 1000 feet of distance between source and load this equates to a little over 750 pounds of copper for the whole system), it is still less than the 1000+ pounds of copper required for a single-phase system delivering the same power (30 kW) at the same voltage (120 volts conductor-to-conductor).

One distinct advantage of a Δ-connected system is its lack of a neutral wire. With a Y-connected system, a neutral wire was needed in case one of the phase loads were to fail open (or be turned off), in order to keep the phase voltages at the load from changing. This is not necessary (or even possible!) in a Δ-connected circuit. With each load phase element directly connected across a respective source phase winding, the phase voltage will be constant regardless of open failures in the load elements.

Perhaps the greatest advantage of the Δ-connected source is its fault tolerance. It is possible for one of the windings in a Δ-connected three-phase source to fail open without affecting load voltage or current!

The only consequence of a source winding failing open for a Δ-connected source is increased phase current in the remaining windings. Compare this fault tolerance with a Y-connected system suffering an open source winding:

With a Δ-connected load, two of the resistances suffer reduced voltage while one remains at the original line voltage, 208. A Y-connected load suffers an even worse fate with the same winding failure in a Y-connected source:

In this case, two load resistances suffer reduced voltage while the third loses supply voltage completely! For this reason, Δ-connected sources are preferred for reliability. However, if dual voltages are needed (e.g. 120/208) or preferred for lower line currents, Y-connected systems are the configuration of choice.

• REVIEW:
• The conductors connected to the three points of a three-phase source or load are called lines.
• The three components comprising a three-phase source or load are called phases.
• Line voltage is the voltage measured between any two lines in a three-phase circuit.
• Phase voltage is the voltage measured across a single component in a three-phase source or load.
• Line current is the current through any one line between a three-phase source and load.
• Phase current is the current through any one component comprising a three-phase source or load.
• In balanced "Y" circuits, line voltage is equal to phase voltage times the square root of 3, while line current is equal to phase current.
• 
• In balanced Δ circuits, line voltage is equal to phase voltage, while line current is equal to phase current times the square root of 3.
• 
• Δ-connected three-phase voltage sources give greater reliability in the event of winding failure than Y-connected sources. However, Y-connected sources can deliver the same amount of power with less line current than Δ-connected sources.

Three-phase power systems

Three-phase power systems

Split-phase power systems achieve their high conductor efficiency and low safety risk by splitting up the total voltage into lesser parts and powering multiple loads at those lesser voltages, while drawing currents at levels typical of a full-voltage system. This technique, by the way, works just as well for DC power systems as it does for single-phase AC systems. Such systems are usually referred to as three-wire systems rather than split-phase because "phase" is a concept restricted to AC.

But we know from our experience with vectors and complex numbers that AC voltages don't always add up as we think they would if they are out of phase with each other. This principle, applied to power systems, can be put to use to make power systems with even greater conductor efficiencies and lower shock hazard than with split-phase.

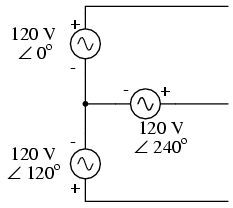
Suppose that we had two sources of AC voltage connected in series just like the split-phase system we saw before, except that each voltage source was 120^o out of phase with the other:

Since each voltage source is 120 volts, and each load resistor is connected directly in parallel with its respective source, the voltage across each load must be 120 volts as well. Given load currents of 83.33 amps, each load must still be dissipating 10 kilowatts of power. However, voltage between the two "hot" wires is not 240 volts (120 ∠ 0^o - 120 ∠ 180^o) because the phase difference between the two sources is not 180^o. Instead, the voltage is:

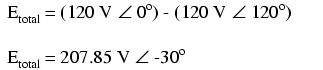
Nominally, we say that the voltage between "hot" conductors is 208 volts (rounding up), and thus the power system voltage is designated as 120/208.

If we calculate the current through the "neutral" conductor, we find that it is not zero, even with balanced load resistances. Kirchhoff's Current Law tells us that the currents entering and exiting the node between the two loads must be zero:

So, we find that the "neutral" wire is carrying a full 83.33 amps, just like each "hot" wire.

Note that we are still conveying 20 kW of total power to the two loads, with each load's "hot" wire carrying 83.33 amps as before. With the same amount of current through each "hot" wire, we must use the same gage copper conductors, so we haven't reduced system cost over the split-phase 120/240 system. However, we have realized a gain in safety, because the overall voltage between the two "hot" conductors is 32 volts lower than it was in the split-phase system (208 volts instead of 240 volts).

The fact that the neutral wire is carrying 83.33 amps of current raises an interesting possibility: since it's carrying current anyway, why not use that third wire as another "hot" conductor, powering another load resistor with a third 120 volt source having a phase angle of 240^o? That way, we could transmit more power (another 10 kW) without having to add any more conductors. Let's see how this might look:

A full mathematical analysis of all the voltages and currents in this circuit would necessitate the use of a network theorem, the easiest being the Superposition Theorem. I'll spare you the long, drawn-out calculations because you should be able to intuitively understand that the three voltage sources at three different phase angles will deliver 120 volts each to a balanced triad of load resistors. For proof of this, we can use SPICE to do the math for us:

120/208 polyphase power system  
v1 1 0 ac 120 0 sin     
v2 2 0 ac 120 120 sin   
v3 3 0 ac 120 240 sin   
r1 1 4 1.44     
r2 2 4 1.44     
r3 3 4 1.44     
.ac lin 1 60 60 
.print ac v(1,4) v(2,4) v(3,4)  
.print ac v(1,2) v(2,3) v(3,1)
.print ac i(v1) i(v2) i(v3)  
.end    

VOLTAGE ACROSS EACH LOAD
freq        v(1,4)      v(2,4)      v(3,4)   
6.000E+01   1.200E+02   1.200E+02   1.200E+02

VOLTAGE BETWEEN "HOT" CONDUCTORS
freq        v(1,2)      v(2,3)      v(3,1)  
6.000E+01   2.078E+02   2.078E+02   2.078E+02 

CURRENT THROUGH EACH VOLTAGE SOURCE
freq        i(v1)       i(v2)       i(v3)     
6.000E+01   8.333E+01   8.333E+01   8.333E+01

Sure enough, we get 120 volts across each load resistor, with (approximately) 208 volts between any two "hot" conductors and conductor currents equal to 83.33 amps. At that current and voltage, each load will be dissipating 10 kW of power. Notice that this circuit has no "neutral" conductor to ensure stable voltage to all loads if one should open. What we have here is a situation similar to our split-phase power circuit with no "neutral" conductor: if one load should happen to fail open, the voltage drops across the remaining load(s) will change. To ensure load voltage stability in the even of another load opening, we need a neutral wire to connect the source node and load node together:

So long as the loads remain balanced (equal resistance, equal currents), the neutral wire will not have to carry any current at all. It is there just in case one or more load resistors should fail open (or be shut off through a disconnecting switch).

This circuit we've been analyzing with three voltage sources is called a polyphase circuit. The prefix "poly" simply means "more than one," as in "polytheism" (belief in more than one deity), polygon" (a geometrical shape made of multiple line segments: for example, pentagon and hexagon), and "polyatomic" (a substance composed of multiple types of atoms). Since the voltage sources are all at different phase angles (in this case, three different phase angles), this is a "polyphase" circuit. More specifically, it is a three-phase circuit, the kind used predominantly in large power distribution systems.

Let's survey the advantages of a three-phase power system over a single-phase system of equivalent load voltage and power capacity. A single-phase system with three loads connected directly in parallel would have a very high total current (83.33 times 3, or 250 amps:

This would necessitate 3/0 gage copper wire (very large!), at about 510 pounds per thousand feet, and with a considerable price tag attached. If the distance from source to load was 1000 feet, we would need over a half-ton of copper wire to do the job. On the other hand, we could build a split-phase system with two 15 kW, 120 volt loads:

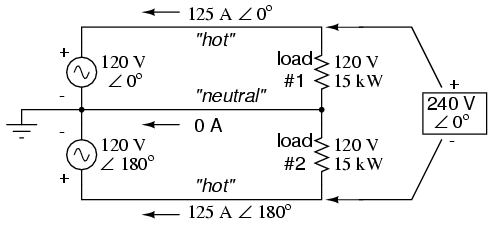
Our current is half of what it was with the simple parallel circuit, which is a great improvement. We could get away with using number 2 gage copper wire at a total mass of about 600 pounds, figuring about 200 pounds per thousand feet with three runs of 1000 feet each between source and loads. However, we also have to consider the increased safety hazard of having 240 volts present in the system, even though each load only receives 120 volts. Overall, there is greater potential for dangerous electric shock to occur.

When we contrast these two examples against our three-phase system, the advantages are quite clear. First, the conductor currents are quite a bit less (83.33 amps versus 125 or 250 amps), permitting the use of much thinner and lighter wire. We can use number 4 gage wire at about 125 pounds per thousand feet, which will total 500 pounds (four runs of 1000 feet each) for our example circuit. This represents a significant cost savings over the split-phase system, with the additional benefit that the maximum voltage in the system is lower (208 versus 240).

One question remains to be answered: how in the world do we get three AC voltage sources whose phase angles are exactly 120^o apart? Obviously we can't center-tap a transformer or alternator winding like we did in the split-phase system, since that can only give us voltage waveforms that are either in phase or 180^o out of phase. Perhaps we could figure out some way to use capacitors and inductors to create phase shifts of 120^o, but then those phase shifts would depend on the phase angles of our load impedances as well (substituting a capacitive or inductive load for a resistive load would change everything!).

The best way to get the phase shifts we're looking for is to generate it at the source: construct the AC generator (alternator) providing the power in such a way that the rotating magnetic field passes by three sets of wire windings, each set spaced 120^o apart around the circumference of the machine:

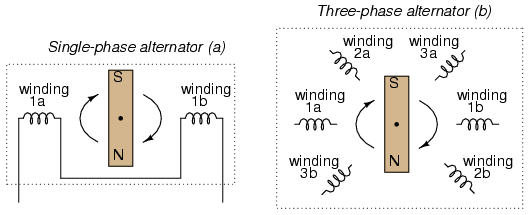
Together, the six "pole" windings of a three-phase alternator are connected to comprise three winding pairs, each pair producing AC voltage with a phase angle 120^o shifted from either of the other two winding pairs. The interconnections between pairs of windings (as shown for the single-phase alternator: the jumper wire between windings 1a and 1b) have been omitted from the three-phase alternator drawing for simplicity.

In our example circuit, we showed the three voltage sources connected together in a "Y" configuration (sometimes called the "star" configuration), with one lead of each source tied to a common point (the node where we attached the "neutral" conductor). The common way to depict this connection scheme is to draw the windings in the shape of a "Y" like this:

The "Y" configuration is not the only option open to us, but it is probably the easiest to understand at first. More to come on this subject later in the chapter.

• REVIEW:
• A single-phase power system is one where there is only one AC voltage source (one source voltage waveform).
• A split-phase power system is one where there are two voltage sources, 180^o phase-shifted from each other, powering a two series-connected loads. The advantage of this is the ability to have lower conductor currents while maintaining low load voltages for safety reasons.
• A polyphase power system uses multiple voltage sources at different phase angles from each other (many "phases" of voltage waveforms at work). A polyphase power system can deliver more power at less voltage with smaller-gage conductors than single- or split-phase systems.
• The phase-shifted voltage sources necessary for a polyphase power system are created in alternators with multiple sets of wire windings. These winding sets are spaced around the circumference of the rotor's rotation at the desired angle(s).